The research of the group connects different subareas of dynamical systems theory, connecting aspects of pure and applied mathematics. The unifying theme concerns the qualitative change of dynamical systems under perturbations and parameter changes, occuring in many fields such as climate science, neuroscience, chemistry or social sciences.

Mathematical subjects investigated within the group:

• Random dynamical systems
• Random attractors and bifurcations
• Ergodic theory and homogenization
• Fast-slow systems
• Geometric singular perturbation theory and geometric blow up
• Relation between continuous-time and discrete-time dynamics
• Stochastic models in population dynamics, game theory and chemical reaction networks

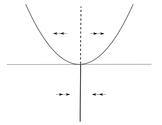
Chaotic random attractor from a stochastic Hopf bifurcation	Pitchfork bifurcation diagram	Snychronization of stochastic trajectories driven by the same noise